Shock Capturing using the Meshless Method

One of the main challenges in astrophysical hydrodynamic simulations is resolving the huge dynamical range of spatial scales, while retaining accurate and physically meaningful results. Astrophysical hydrodynamics usually relies on two numerical approaches: the highly dynamic SPH (Smoothed Particle Hydrodynamics) method and the more accurate but resolution limited grid method. Therefore, an ideal case would be a method with the dynamic range of SPH codes which preserves the shock capturing ability of grid based codes.
One solution to this problem may lie in the use of the relatively new Meshless Finite method (Lanson & Vila, 2008a,b; Gaburov & Nitadori, 2011). In this method, the particles are not static but move around as in SPH. However, when the values of conserved variables (mass, momentum etc.) are updated, a Riemann problem is solved between each pair of neighbouring particles, similar to the way how it is calculated in grid codes.
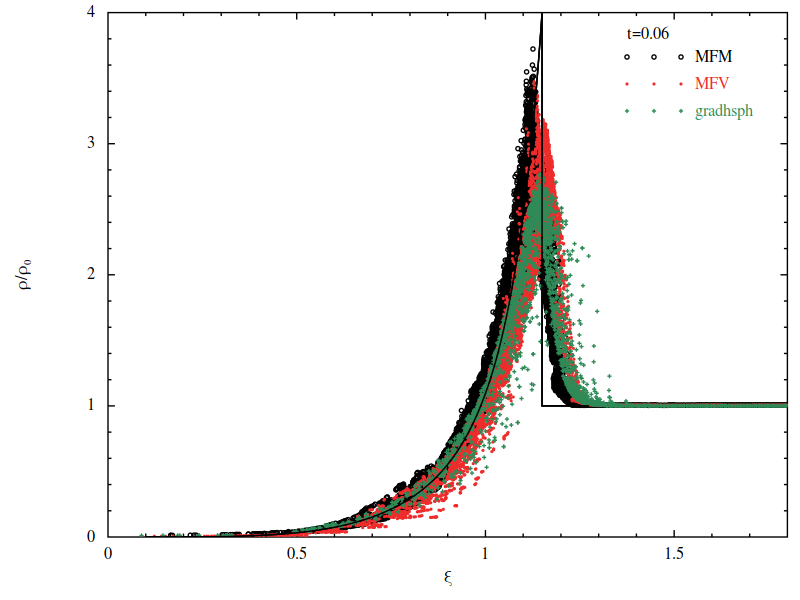
This method retains the dynamical range of SPH, while having better shock capturing abilities among other advantages. The figure compares a standard SPH with our implementation of two flavours of the meshless finite method applied on a Sedov blast wave test.
The Sedov blast wave test is a demanding hydrodynamic test that models the Sedov-Taylor phase of the expansion of a supernova in an ambient interstellar medium. Computationally, it is performed by having a highly energetic core expand in a uniform medium. The core expands with a strong shock front, where the shock profile is given by a self similar solution. The figure shows that both flavours of the meshless method capture the shock much better than SPH of the same resolution.