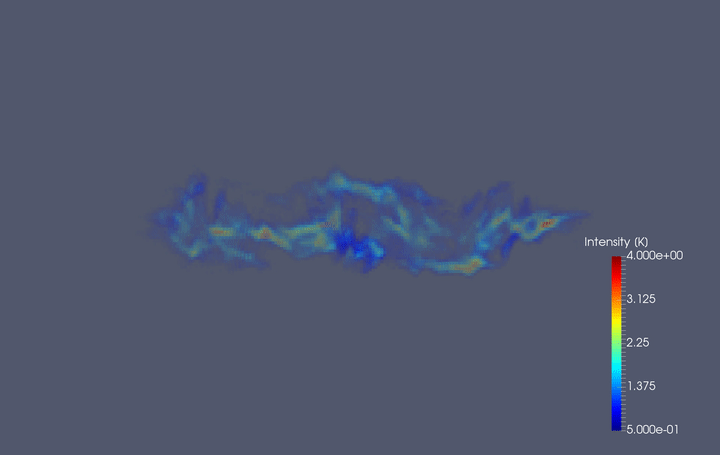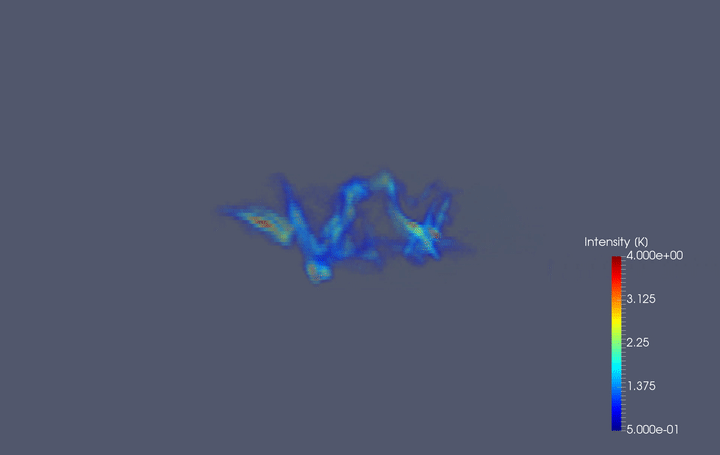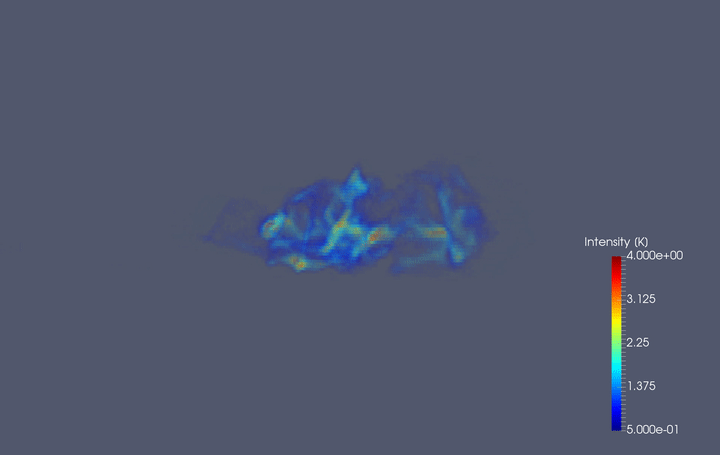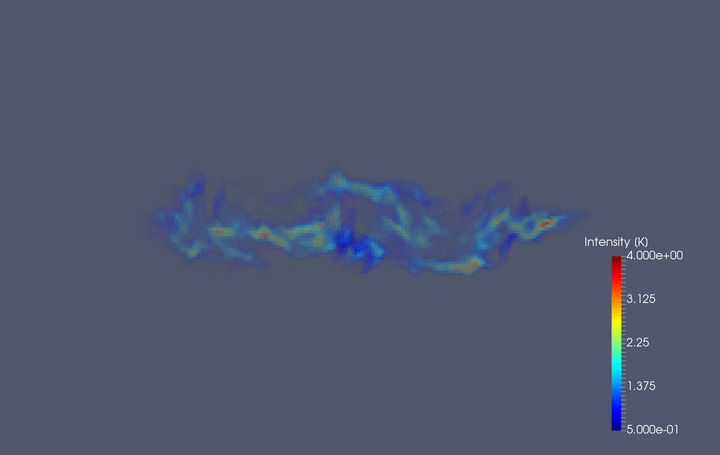
Synthetic observations of fibrous filaments: the problems of mapping from PPV to PPP

We present synthetic C$^{18}$O observations of fragmenting filaments produced with the moving-mesh hydrodynamic code AREPO. In the simulations we find that numerous continuous volume density sub-filaments form inside the parent filament due to accretion-driven turbulence. The synthetic observations of these simulations reveal the existence of velocity-coherent filamentary substructures which are similar to the 'fibres' detected in Taurus.
However, we show that while there exist filamentary substructures in both the simulations and in the synthetic observations, these are not the same structures. Only 50$\%$ of structures identified in position-position-velocity (PPV) space are free from line-of-sight confusion and form a physically continuous structure in position-position-position (PPP) space. Those PPV structures which are not affected by line-of-sight confusion do not correspond to the sub-filaments seen in the simulations. Sub-filaments identified as continuous density structures in PPP are imprinted with large velocity gradients resulting from the formation of the parent filament, in addition to the internal turbulence driven by accretion. This leads to the individual sub-filaments existing over a wide range of velocities and split into multiple velocity coherent structures due to shocks.
These results suggest that properties of velocity coherent structures do not accurately describe the underlying substructure within a filament. Furthermore, the condition that a filamentary structure be velocity coherent and distinct in PPV is not a sufficient condition to guarantee that it is a continuous and distinct structure in PPP.