Two-dimensional Gratings

The simplest type of two-dimensional grating is produced by orthogonally overlaying two one-dimensional structures. This results in a rectangular pattern of beams and the grating efficiency equals the product of the two one-dimensional efficiencies.

The design of a more complex two-dimensional grating is much more difficult due to the higher number of independent Fourier coefficients. While the one-dimensional or rectangular gratings only require the optimization of $N$ or $2N$ coefficients, respectively, the general two-dimensional grating uses $N^2$ coefficients. This high number of coefficients makes it very inefficient to randomly search for starting values as described above.

For this reason, we chose a slightly different approach for the optimization of the two-dimensional gratings. Modeling the grating as a beam combiner, we summed the fields from the desired number of diffraction orders incident on the grating as plane waves in the grating plane. The combined field, ( i.e. the inverse Fourier transform of the diffraction pattern), then varies in both amplitude and phase over the grating. Since we want to design a pure phase grating, we optimize the phases in the incident waves to minimize the amplitude variation over the grating surface. The Fourier series expansion of the resulting phase variation is then taken as the starting values for the grating coefficients $a_n$. The further optimization of the grating is identical to the one-dimensional case.

This method is very efficient in producing good starting values for the optimization. However, since the parameter search is less complete than in our initial method, it is less obvious that it converges to the best possible grating. If, for instance, the optimum phase structure has a dynamic range of more than $2\pi$, the random parameter search will still model it accurately, whereas the second method introduces phase wraps. Although these $2\pi$ phase wraps are physically irrelevant, they degrade the quality of the Fourier series expansion and make it more difficult to manufacture the gratings.

Figure 5: Surface topology of the unit cell for a Fourier grating producing a 2-4-2 arrangement of beams. 13 Fourier coefficients have been used in the optimization of each direction. Contour levels are from -1.5 to 1.5 radians in steps of 0.25 radians. The theoretical efficiency of this structure is 0.84.

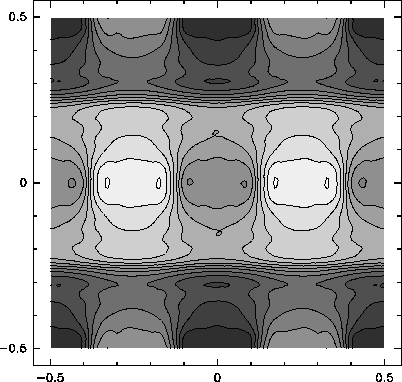As an example for a non-trivial two-dimensional structure, we show in Fig. 5 a grating, which produces 8 identical beams arranged in a 2-4-2 pattern. This grating is required for the splitting of the local oscillator in a 4$\times$4 array receiver, which uses two interleaved 8 pixel subarrays to increase the beam packaging density. With this 13$\times$13 coefficient Fourier grating we reach a diffraction efficiency of approximately 84%.