Asymmetric Gratings

The problem of suppressing the even diffraction orders in a symmetric 4-beam grating may be circumvented by choosing an asymmetric configuration. Can this yield higher diffraction efficiencies?

Figure 4: Transition from a symmetric grating to an asymmetric grating. Adding a phase gradient (dotted line) to the symmetric structure (left hand panels) shifts the diffraction pattern by half a beam (right hand panels). Replacing the $+\pi$ phase steps by $-\pi$ steps results in the optimized structure for the asymmetric grating (solid line). The net effect of this procedure is the replacement of the step function underlying the symmetric grating by a -0.5$^{\rm th}$ order blaze function.

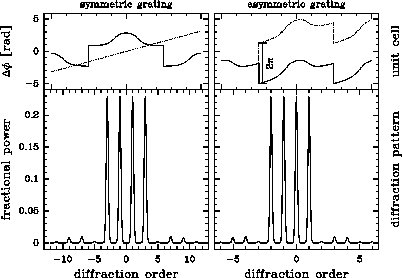
If we choose the diffraction orders -2, -1, 0, and +1 for the four beams, our optimization algorithm converges for $N\to \infty$ to exactly the same maximum efficiency. The resulting grating structure is identical to the optimum structure for the symmetric case (Fig. 1) except that the periodic step function is replaced by a blaze function with a blaze angle corresponding to the -0.5$^{\rm th}$ diffraction order (Fig. 4). The diffraction patterns are also identical except for a relative shift of one diffraction order. The unit cell of the asymmetric grating has only half the size of the symmetric unit cell. Therefore, the diffraction orders are more widely spaced by a factor of two, which keeps the angular spacing between the four beams constant.

In a similar fashion, other sets of equally spaced diffraction orders may be chosen, and the required grating can be constructed from the basic symmetric 4-beam grating.